3DNLG-10: Elastic-plastic behavior of a stiffened cylindrical panel under compressive end load

This problem provides evidence that Abaqus can reproduce the result from the benchmark defined by NAFEMS and cited as the reference solution.

This page discusses:

Elements tested
Problem description
Reference solution
Results and discussion
Response predicted by Abaqus
Input files

ProductsAbaqus/Standard

Elements tested

S3
S3R
S4
S4R
S4R5
S8R
S8R5
S9R5

STRI3
STRI65

Problem description

Model:

There is an initial imperfection in both the cylindrical panel and the stiffener.

Cylindrical panel: $Δ R = 0.569 \sin \frac{(θ π^{2} Z)}{7200}$ , where −9 $\leq θ \leq$ 9 and 0 $\leq Z \leq$ 200.

Stiffener: $Δ z = - 0.3 \sin \frac{(π x)}{400} \sin \frac{(π y)}{20}$ , where 0 $\leq x \leq$ 200 and 0 $\leq y \leq$ 10.

Material:

Young's modulus = 2.1 × 10⁵, Poisson's ratio = 0.3, yield stress = 350.

Boundary conditions:

Tangential symmetry along edges 1 and 2 at $θ$ = $\pm$ 9° ( $U_{θ} = θ_{R} = θ_{Z} = 0$ ). Simply supported at end A for panel: ( $U_{R} = U_{θ} = θ_{R} = θ_{Z} = 0$ ), for stiffener: ( $U_{y} = U_{z} = θ_{x} = 0$ ). Symmetry at $Z = L / 2$ for panel: ( $U_{Z} = θ_{R} = θ_{θ} = 0$ ), for stiffener: ( $U_{x} = θ_{y} = θ_{z} = 0$ ).

Loading:

Compressive, evenly distributed edge load. The RIKS algorithm is used to increment the load until the global z-displacement at point D exceeds 0.2.

Reference solution

This is a test recommended by the National Agency for Finite Element Methods and Standards (U.K.): Test 3DNLG-10 from NAFEMS Publication R0024 “A Review of Benchmark Problems for Geometric Non-linear Behaviour of 3D Beams and Shells (SUMMARY).”

The published results of this problem were obtained with Abaqus. Thus, a comparison of Abaqus and NAFEMS results is not an independent verification of Abaqus. The NAFEMS study includes results from other sources for comparison that may provide a basis for verification of this problem.

Results and discussion

In the following table, limit points 1 and 2 correspond to the peak and local minimum, respectively, of the load-displacement curve. To produce results comparable to those for S8R5/S9R5, the first-order elements require a finer mesh with half the nodal spacing in the curved direction of the panel. The limit loads for the thick shell element S8R are noticeably higher than others in this thin shell application, even when a fine mesh with the same nodal spacing as that used for the first-order elements is generated.


Element	Limit point 1		Limit point 2
	End load	$U_{z}$ at D	End load	$U_{z}$ at D
S3/S3R	23357	0.167	15622	0.138
S4	23505	0.168	15097	0.135
S4R	23621	0.168	15126	0.136
S4R5	23540	0.168	15074	0.136
S8R	25318	0.180	16964	0.146
S8R5	23764	0.169	15453	0.138
S9R5	23789	0.170	15460	0.138
STRI3	22993	0.165	14809	0.134
STRI65	22534	0.161	15048	0.133

Response predicted by Abaqus

Similar load-displacement curves are obtained for all the test cases. The response predicted using S8R5 elements is shown below.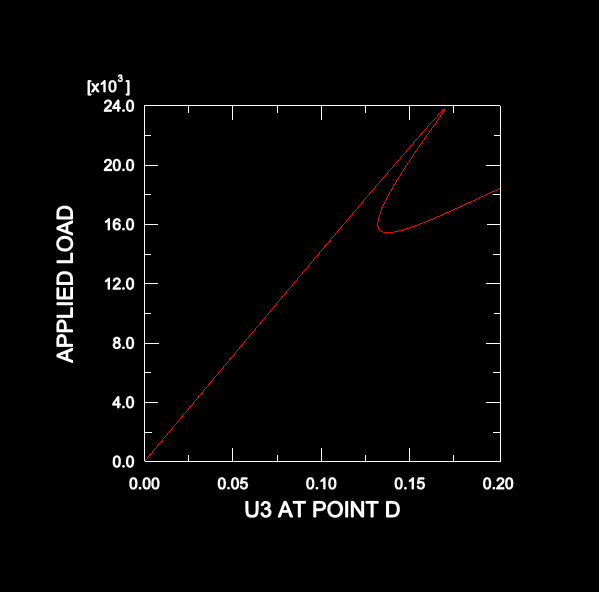

Input files

n3g10f3x.inp: S3/S3R elements.
n3g10e4x.inp: S4 elements.
n3g10f4x.inp: S4R elements.
n3g1054x.inp: S4R5 elements.
n3g1068x.inp: S8R elements.
n3g1058x.inp: S8R5 elements.
n3g1059x.inp: S9R5 elements.
n3g1063x.inp: STRI3 elements.
n3g1056x.inp: STRI65 elements.