Sources of Nonlinearity

This class of nonlinear behavior stems from the nonlinear relationship between the stress and strain.

Most metals have a fairly linear stress/strain relationship at low strain values; but at higher strains the material yields, at which point the response becomes nonlinear and irreversible. Several factors can affect the stress-strain relationship, such as:

• Load history: plasticity problems
• Load duration: creep analysis, viscoelasticity
• Temperature: thermoplasticity

A typical stress-strain diagram of a ductile material model is shown in the figure below. The elastic range of the material ends when the stress has reached the yielding point. Once the material has reached the yielding point, permanent deformation, which is non-reversible, begins to develop. As the tensile stress increases, the plastic deformation is characterized by a strain hardening region, necking region, and finally fracture.

Rubber materials can be approximated by a nonlinear, reversible (elastic) response. A typical stress-strain curve of a rubber material is shown below.

This class of nonlinear behavior occurs if the boundary conditions change during the analysis. Boundary nonlinearities occur in manufacturing processes, such as forging and stamping.

An example of boundary nonlinearity is blowing a sheet of material into a mold. The sheet expands relatively easily under the applied pressure until it begins to contact the mold. From then on, because of the change in boundary conditions, the pressure must be increased to continue forming the sheet.

Consider the cantilever beam that deflects under an applied load until it hits a "stop."

The vertical deflection of the tip is linearly related to the load (if the deflection is small) until it contacts the stop. There is then a sudden change in the boundary condition at the tip of the beam, preventing any further vertical deflection; hence, the response of the beam is no longer linear.

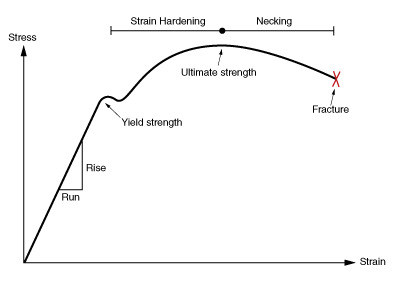
The third source of nonlinearity is related to changes in the geometry of the structure during the analysis. Geometric nonlinearities occur whenever the magnitude of the displacements affects the response of the structure. This might be caused by:

• Large deflections or rotations
• Snap through behavior
• Initial stresses or load stiffening

In general, large displacements can cause the structure to respond in a stiffening or softening manner as shown in the figure below.

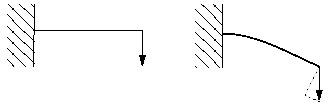
For example, consider a cantilever beam loaded vertically at the tip.

If the tip deflection is small, the analysis can be considered as being approximately linear. However, if the tip deflections are large, the shape of the structure and, hence, its stiffness changes. In addition, if the load does not remain perpendicular to the beam, the action of the load on the structure changes significantly.

As the cantilever beam deflects, the load can be resolved into a component perpendicular to the beam and a component acting along the length of the beam. Both of these effects contribute to the nonlinear response of the cantilever beam (i.e., the changing of the beam's stiffness as the load it carries increases).

One would expect large deflections and rotations to have a significant effect on the way that structures carry loads. However, displacements do not necessarily have to be large relative to the dimensions of the structure for geometric nonlinearity to be important.

Consider the "snap through" under applied pressure of a large panel with a shallow curve.

In this example there is a dramatic change in the stiffness of the panel as it deforms. As the panel "snaps through," the stiffness becomes negative. Thus, although the magnitude of the displacements, relative to the panel's dimensions, is quite small, there is significant geometric nonlinearity in the simulation, which must be taken into consideration.