Fluid-filled spherical shell response to a planar step wave

This example illustrates the use of Abaqus/Explicit to model the interaction between a fluid-filled spherical elastic shell and a planar step wave. The results obtained using Abaqus/Explicit are compared with those obtained independently using the Doubly Asymptotic Approximation (Geers (1978), Abaqus/USA 6.1). This problem has been solved analytically by Zhang and Geers (1993).

This page discusses:

ProductsAbaqus/Explicit

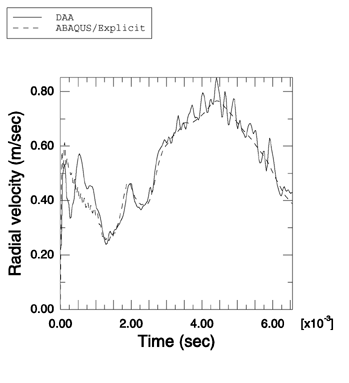
Problem description

This problem models the interaction between a water-backed spherical elastic shell and a weak planar step shock wave with a maximum pressure of 1 MPa. In contrast to the solution from Zhang and Geers, engineering material parameters for the fluid and solid media are used. The sphere has a radius of 1 m and a thickness of 0.01 m. The sphere is made of steel with a density of 7677 kg/m3, a Young's modulus of 210.0 GPa, and a Poisson's ratio of 0.3. The fluid is water with a density of 997 kg/m3, in which the speed of sound is 1524 m/s. An axisymmetric model is used for this analysis. The spherical shell is modeled with SAX1 elements, while the enclosed and surrounding fluid is modeled with ACAX4R elements. The inner semicircle that bounds the fluid region is coincident with the shell, and the outer semicircle has a radius of 3 m. A spherical nonreflective boundary condition is imposed on the outer semicircle using surface impedance. The fluid response is coupled to that of the structure using a tie constraint on both sides of the spherical shell, with the shell surfaces as the main surfaces. The fluid-solid system is excited by a planar step wave applied at the point where the semicircular shell intersects the axis of symmetry using incident wave loading. A linear bulk viscosity parameter of 0.2 and a quadratic bulk viscosity parameter of 1.2 are used.

Results and discussion

The results from Abaqus/Explicit show good qualitative comparison with those in the referenced literature. We also compare the numerical values for radial velocities at the leading and trailing points on the shell obtained using Abaqus/Explicit with those obtained using Abaqus/USA 6.1. As shown in Figure 1 and Figure 2, the results agree closely.

References

  1. Geers,  T., “Doubly Asymptotic Approximations for Transient Motions of Submerged Structures,” Journal of the Acoustical Society of America, vol. 64, pp. 1500–1508, 1978.
  2. Zhang,  P.,  and T. L. Geers, “Excitation of a Fluid-Filled, Submerged Elastic Shell by a Transient Acoustic Wave,” Journal of the Acoustical Society of America, vol. 93, pp. 696–705, 1993.

Figures

Figure 1. Comparison of the radial velocity at the leading point on the spherical shell obtained with the Doubly Asymptotic Approximation method and with Abaqus/Explicit.

Figure 2. Comparison of the radial velocity at the trailing point on the spherical shell obtained with the Doubly Asymptotic Approximation method and with Abaqus/Explicit.