Transient loading of a viscoelastic bushing

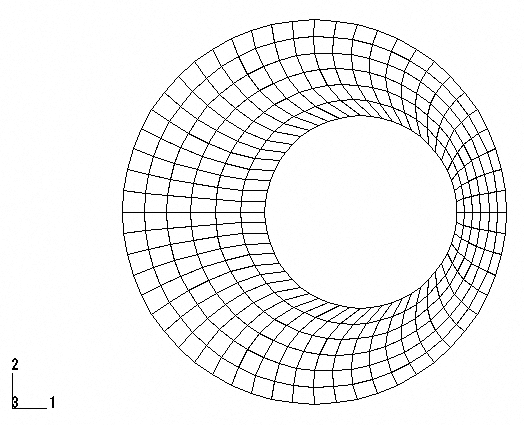
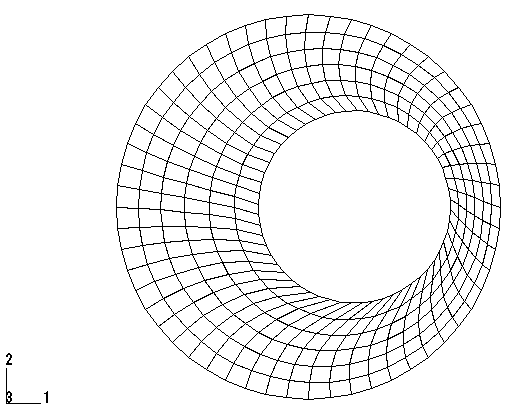
The viscoelastic bushing has an inner radius of 12.7 mm (0.5 in) and an outer radius of 25.4 mm (1.0 in). We assume that the bushing is long enough for plane strain deformation to occur. The problem is modeled with first-order reduced-integration elements (CPE4R). The mesh is regular, consisting of 6 elements radially, repeated 56 times to cover the 360° span in the hoop direction. The mesh is shown in Figure 1. No mesh convergence studies have been performed.

The fixed outer body is modeled by fixing both displacement components at all the outside nodes. The nodes in the inner boundary of the bushing are connected, using a kinematic coupling constraint, to a node located in the center of the model. This node, thus, defines the inner shaft as a rigid body.

The material model is not defined from any particular physical material.

The instantaneous behavior of the viscoelastic material is defined by hyperelastic properties. A polynomial model with $N=$1 (a Mooney-Rivlin model) is used for this, with the constants $C_{10}=$ 27.56 MPa (4000 psi), $C_{01}=$ 6.89 MPa (1000 psi), and $D_1=$ 0.0029 MPa⁻¹ (0.00002 psi⁻¹).

The viscous behavior is modeled by a time-dependent shear modulus, $G_R\left(t\right)$, and a time-dependent bulk modulus, $K_R\left(t\right)$, each of which is expanded in a Prony series in terms of the corresponding instantaneous modulus,

The relative moduli ${\overline{g}}_i^P$ and ${\overline{k}}_i^P$ and time constants ${\tau }_i$ are

This model results in an initial instantaneous Young's modulus of 206.7 MPa (30000 psi) and Poisson's ratio of 0.45. It relaxes pressures faster than shear stresses.

The analysis is done in four steps. The first step is a preload of 222.4 kN (50000 lbs) applied in the x-direction to the node in the center of the model in 0.001 sec with a static procedure (Static Stress Analysis). The static procedure does not allow viscous material behavior, so this response is purely elastic. During the second step the load stays constant and the material is allowed to creep for 1 sec by using the quasi-static procedure (Quasi-Static Analysis). Since 1 sec is a long time compared with the material time constants, the solution at that time should be close to steady state. The accuracy of the automatic time incrementation during creep response can be controlled. This accuracy tolerance is an upper bound on the allowable error in the creep strain increment in each time increment. It is chosen as 5 × 10⁻⁴, which is small compared to the elastic strains. The third step is another static step. Here the loading is a torque of 1129.8 N-m (10000 lb-in) applied in 0.001 sec. The fourth step is another quasi-static step with a time period of 1 sec.