As the strains are so large, the results should compare very closely with
the exact, rigid-plastic solution of Prager and Hodge (1951). That exact
solution gives the stresses as follows:
where k is the yield stress in pure shear
(
times the yield stress in pure tension); a0 is
the initial inside radius; b0 is the initial
outside radius; r0 is the radius, in the
initial configuration, of the material point at which the stresses are being
calculated; and
is the current value of the inside radius. The form of the solution shows that
we need only compare the radial stress, since the other stresses are obtained
directly from that component.
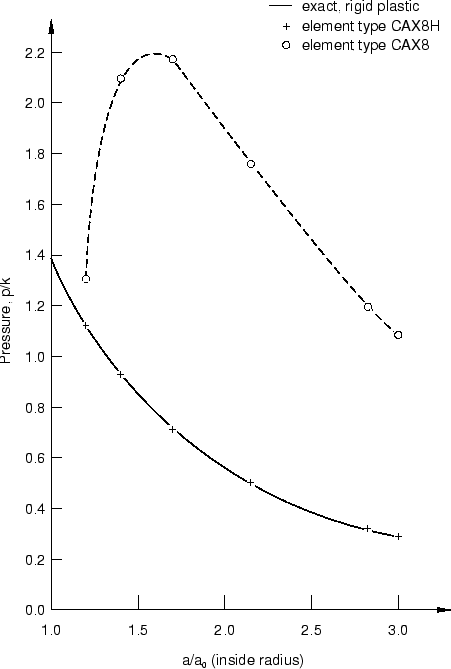
The results for the axisymmetric element models are summarized in
Figure 2
and
Figure 3,
while
Figure 4
and
Figure 5
show the results for the plane strain models.
Figure 2
compares the pressure versus inside radius given by the CAX8H and CAX8 finite element models to that given by the exact, rigid-plastic
solution. All of the axisymmetric models agree very closely with the exact
solution with the exception of that using the fully integrated 8-node (CAX8) element. Recall that the solution is obtained by prescribing the
motion of the inside surface of the cylinder, so the pressure is calculated for
the finite element models from the reaction forces conjugate to these
prescribed displacements.
Figure 3
compares the stress calculated by
Abaqus
at the point initially halfway through the cylinder wall
(
1.5) to the exact, rigid-plastic solution. Again, with the exception of the CAX8 element model, there is excellent agreement with the analytical
solution.
Figure 4
and
Figure 5
show similar results to
Figure 2
and
Figure 3
for the plane strain models. Again, with the exception of the fully integrated
8-node (CPE8) element model, all of the plane strain models show excellent
agreement with the exact, rigid-plastic solution.
The pure displacement 8-node elements (CAX8 and CPE8) give poor results because the strains are calculated directly
from the interpolation functions at each integration point, and the
incompressibility requirement causes a severe oscillation in the mean pressure
stress throughout each element. However, in the hybrid, 8-node elements the
mean pressure stress is interpolated independently, so an accurate value is
obtained for this variable. In addition, the 4-node elements in
Abaqus
are constant strain/stress elements for this case (because these elements are
coded with a constant hoop strain value and use “selective reduced
integration,” in which the volume strain is computed at the centroid only), and
so also provide accurate pressure stress values.
Results for models using the fully integrated versions of axisymmetric and
plane strain elements are shown here to caution the user. With rare exceptions
the fully integrated 8-node quadrilaterals are not as effective as the reduced
integration versions of the same elements; the reduced integration 8-node
quadrilaterals are, hence, almost always recommended over their fully
integrated counterparts. This particular problem gives a dramatic illustration
of a difficulty encountered with full integration in a problem in which the
bulk behavior of the material is very much stiffer than the shear behavior, a
type of behavior commonly encountered.