Near bottom pipeline pull-in and tow

This example verifies that Abaqus correctly simulates the “near-bottom bending” method for installing a pipeline on the seabed floor and the subsequent towing process.

This page discusses:

ProductsAbaqus/StandardAbaqus/Aqua

In the “near-bottom bending” approach chains, typically 5–10 m (20–30 ft) long, are attached to the pipeline at intervals along its length. Their weight then balances the buoyancy devices, which are attached to the pipeline, when the pipeline is lowered to a position about 3 m (10 ft) from the seabed. The pipeline is then winched into position at each end, with the lengths of chain lying on the seabed acting as restraints on the motion and, thus, providing some control over the process. One of the analyses in this example is the prediction of configuration and stress in the pipeline throughout such a “pull-in” process, the usual concern being to accomplish a satisfactory final configuration without buckling or overstressing the pipeline at any time during the installation. As a second near-bottom pipeline installation example, the cable is assumed to remain constant in length and motion is prescribed on the unattached end, thereby simulating a towing process.

During the pull-in or towing process the chains typically take the configuration shown in Figure 1: a catenary between the attachment point and the seabed, with some length along the seabed (this part of the chain may not lie in a straight line along the seabed: its configuration depends on the previous motion). In Abaqus this is idealized as a single anchor block on the seabed, connected to the attachment point by a catenary (Figure 2). When two-dimensional drag chains are used, the model requires the specification of two parameters: the horizontal distance, ls, between the attachment point and the anchor block when the system is slipping (that is, the maximum possible horizontal distance between these points, since the horizontal force is limited by friction) and this maximum frictional force. Typically, ls is chosen as the horizontal distance between the attachment point and a point halfway along the horizontal chain lying on the seabed, while the maximum frictional force is μ⁢w⁢l0, where μ is the friction coefficient, w is the weight of the chain per unit length (in water), and l0 is the length of chain on the seabed in the actual configuration. The three-dimensional drag chains can also be used. In this case the model requires the specification of three parameters: the total length of the chain, the friction coefficient, and the weight per unit length of chain. The total length of the chain is the sum of the length of the chain on the seabed and the suspended length. In addition, for three-dimensional analyses the seabed must be defined using a rigid surface, which must be flat and parallel to the global X–Y plane.

This idealization of the drag chains is usually satisfactory for motions several times larger than typical lengths associated with these chains (l0). For small motions (of order l0) the model is too idealized, and the chain must itself be modeled. Since the majority of installation procedures involve considerable motion, the model is usually adequate.

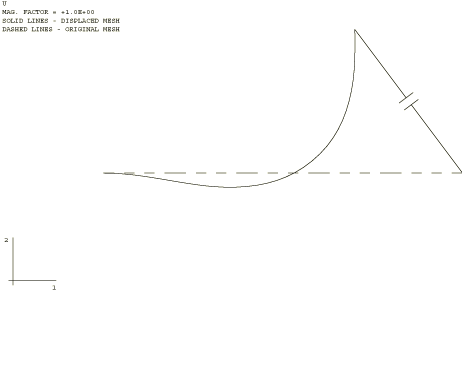
Problem description

The example used here to illustrate the process consists of a pipe of length 304.8 m (1000 ft), with an outer diameter of 228.6 mm (0.75 ft) and a wall thickness of 7.62 mm (0.025 ft). This is a rather slender beam, and for this reason hybrid beam element type B23H is chosen. (The “hybrid” beams are mixed formulation elements designed for use with very slender or very stiff systems.) One end of the pipeline is winched into an anchor point that is initially offset 121.92 m (400 ft) to one side of the pipeline and set back 91.44 m (300 ft) from the end of the pipeline. The other end of the pipeline is assumed to be built in—that is, already fully attached to some rigid fixture, as indicated in Figure 3.

There are six equally spaced drag chains on the pipeline, and so for convenience five elements are used to idealize the pipeline. Drag chains are attached to the nodes. The chain at the end being winched has a mean length at slip, ls, of 7.62 m (25 ft) and requires a force of 556 N (125 lb) to slip. The other chains are all of equal size, with a mean length at slip of 1.524 m (5 ft) and a slip force of 111 N (25 lb).

For comparison purposes the analysis is also performed using three-dimensional drag chains. For this case hybrid beam element type B33H is used, and the z-displacements at all of the beam nodes are restrained to reproduce the two-dimensional case. The equivalent three-dimensional parameters are obtained based on the description outlined in Drag Chains. The chain at the end of the pipe will have a total length lc of 39.9 m (131 ft), a friction coefficient μ of 0.3, and a weight per unit length of 58.2 N/m (4.0 lb/ft). The remaining chains have a total length lc of 7.98 m (26.2 ft), a friction coefficient μ of 0.1, and a weight per unit length of 1455 N/m (100 lb/ft). The height of the beam above the seabed, h, is 3.05 m (10 ft). A cylindrical analytical surface with a fixed reference node is used to simulate the seabed. The reference node is used as the second node of the DRAG3D element to associate the drag chains with the seabed.

The cable is modeled as a spherical gap element, which provides for an inextensible cable supporting tension but no compression. The length of the cable can be changed throughout a step by using a contact interference. This feature is used here to reduce the length to zero over the step and, thus, effect the pull-in.

Material

The pipeline is made of steel, with a Young's modulus of 206.8 GPa (4.32 × 109 lb/ft2). Since the material response is assumed to remain elastic throughout the process, a general beam section is used: with this section Abaqus integrates the elastic section response exactly. If nonlinear material response is involved, numerical integration of the section is required; hence, a beam section should be used instead.

Boundary conditions

For the pull-in analysis the left-hand end of the pipe is assumed to be held rigidly, including full rotational restraint. For the three-dimensional analysis the beam nodes are also restrained in the z-direction to simulate the two-dimensional case. The rigid surface reference node is fully restrained in all six degrees of freedom. The anchor point node is restrained in all directions in this case, since the pull-in is toward a fixed point.

For the near-bottom tow analysis the pipeline is unrestrained for the analysis using DRAG2D elements; however, when DRAG3D elements are used, the pipeline is restrained in the z-direction as described above. The tow is up the y-axis: the anchor point is fixed in the x-direction, and a motion of 304.8 m (1000 ft) is prescribed in the y-direction. This implies that the pipeline has no restraint (and is, therefore, singular) until the drag chain extends sufficiently to stabilize the pipeline. To overcome numerical difficulties in the early stages of the analysis, soft springs are attached to two pipeline nodes. When the system is no longer singular, the solution proceeds smoothly, with the automatic time incrementation algorithm controlling the increment size.

Results and discussion

Both the two-dimensional and three-dimensional drag chain elements produce the same response. The results for the two examples studied are discussed below.

Pull-in analysis

The configuration of the pipeline at the end of the pull-in analysis is shown in Figure 4. It is interesting to notice some swiveling of the pipeline: part of the pipeline moves in the negative y-direction, by as much as 12.2 m (40 ft). Presumably this occurs because of the direction of pull-in and the pointwise resistance to motion provided by the drag chains. It is instructive to contrast this with the results of Pull-in of a pipeline lying directly on the seafloor, where the pull-in of a pipeline lying directly on the seabed is simulated.

Towing analysis

The simulation is complicated in this case by the lack of restraint on the pipeline in its initial configuration. At the start of the analysis, as point A (see Figure 3) moves in the positive y-direction, the pipeline moves slightly in the negative x-direction, and the parts of the pipeline farthest from the cable also move in the negative y-direction. Then, as the analysis proceeds, the pipeline straightens out, taking on the configuration shown in Figure 5 at the end of the prescribed towing motion.

Actual installation processes usually involve considerably more complex winching and alignment histories than those shown here. Such complex histories can be simulated in a series of steps, each specifying a phase of the installation.

Figures

Figure 1. Actual drag chain.

Figure 2. Drag chain model.

Figure 3. Pipeline pull-in and towing problems (boundary condition only for the pull-in analysis).

Figure 4. Final configuration—pipeline pull-in, drag chains.

Figure 5. Final configuration—pipeline tow with drag chains.